

目录
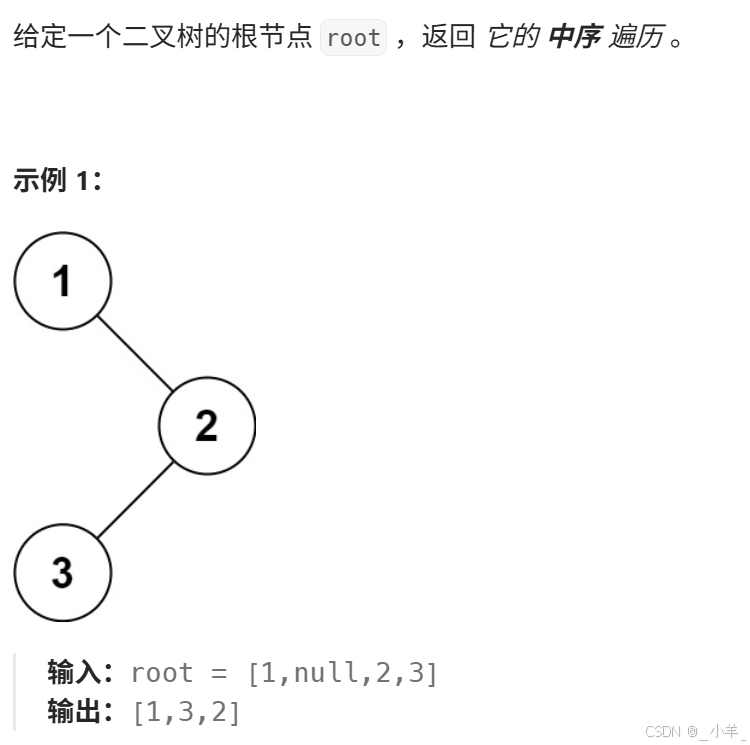
二叉树的中序遍历
- 二叉树的中序遍历
class Solution {vector<int> res;
public:vector<int> inorderTraversal(TreeNode* root) {dfs(root);return res;}void dfs(TreeNode* root){if (root == nullptr) return;dfs(root->left);res.push_back(root->val);dfs(root->right);}
};
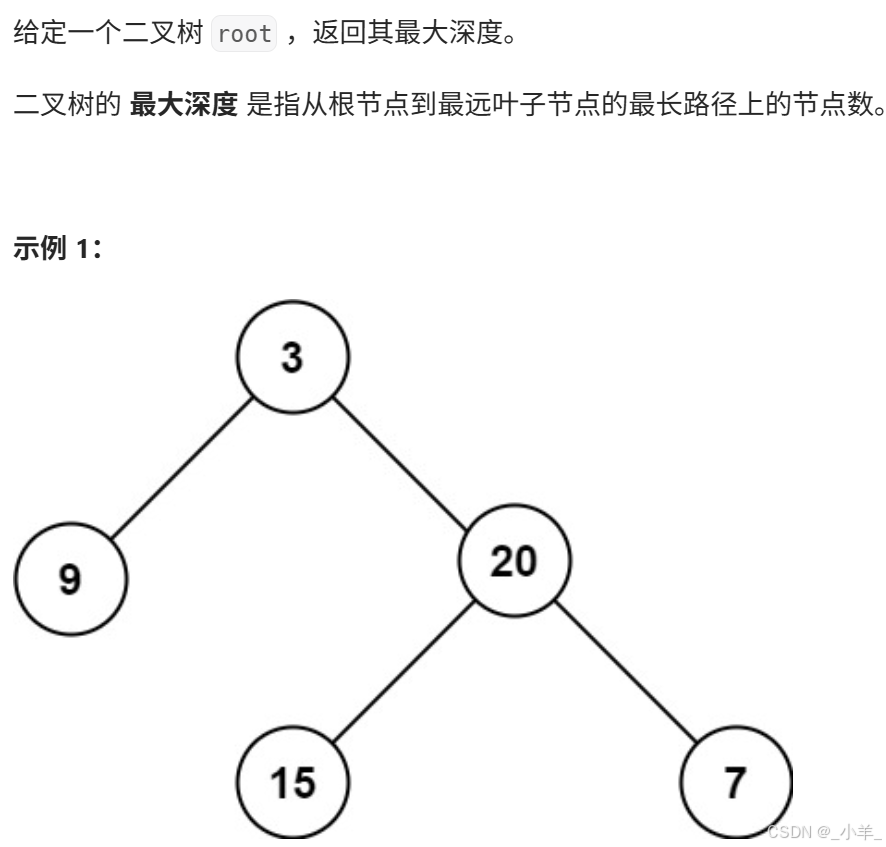
二叉树的最大深度
- 二叉树的最大深度
class Solution {
public:int maxDepth(TreeNode* root) {if (root == nullptr) return 0;int left = maxDepth(root->left);int right = maxDepth(root->right);return left > right ? left + 1 : right + 1;}
};
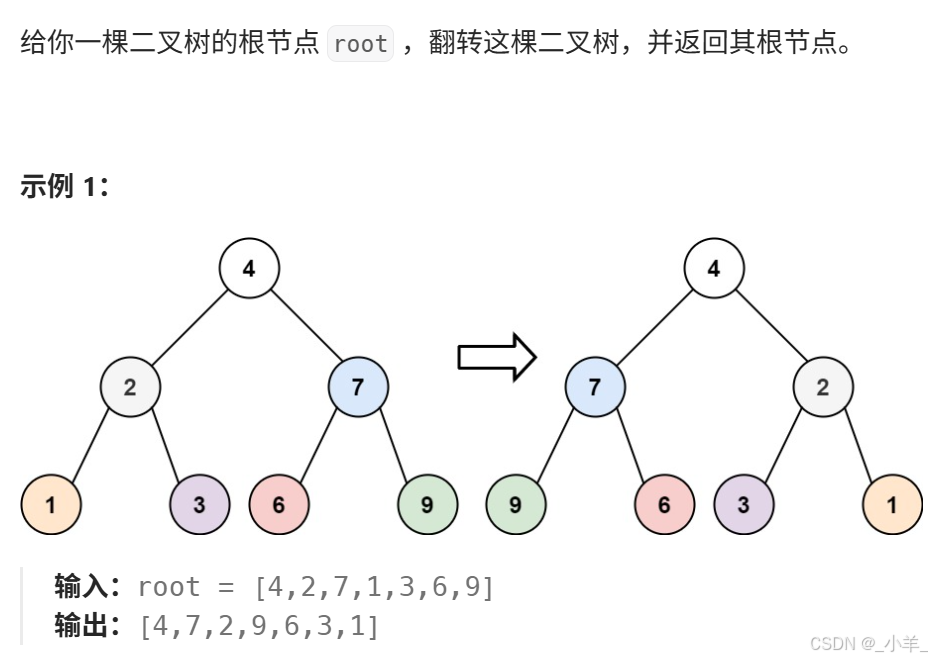
翻转二叉树
- 翻转二叉树
class Solution {
public:TreeNode* invertTree(TreeNode* root) {if (root == nullptr) return nullptr;TreeNode *left = invertTree(root->left);TreeNode *right = invertTree(root->right);root->left = right;root->right = left;return root;}
};
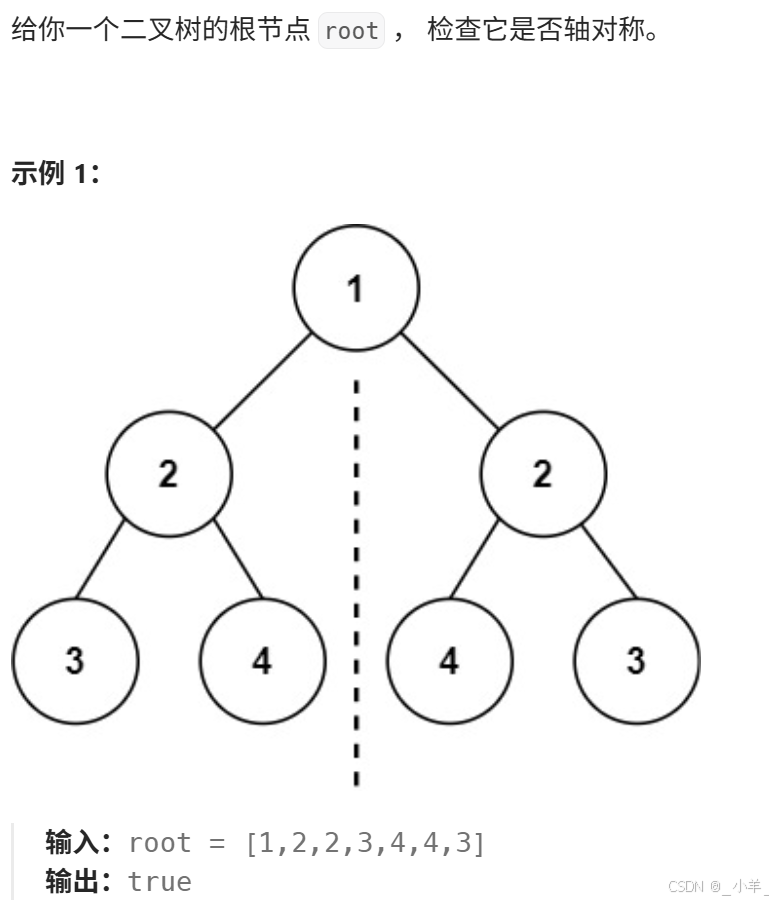
对称二叉树
- 对称二叉树
class Solution {
public:bool isSymmetric(TreeNode* root) {return dfs(root->left, root->right);}bool dfs(TreeNode* left, TreeNode* right){if (left && right){if (left->val != right->val) return false;return dfs(left->left, right->right) && dfs(left->right, right->left);}else if (left != right) return false;else return true;}
};
二叉树的直径
- 二叉树的直径
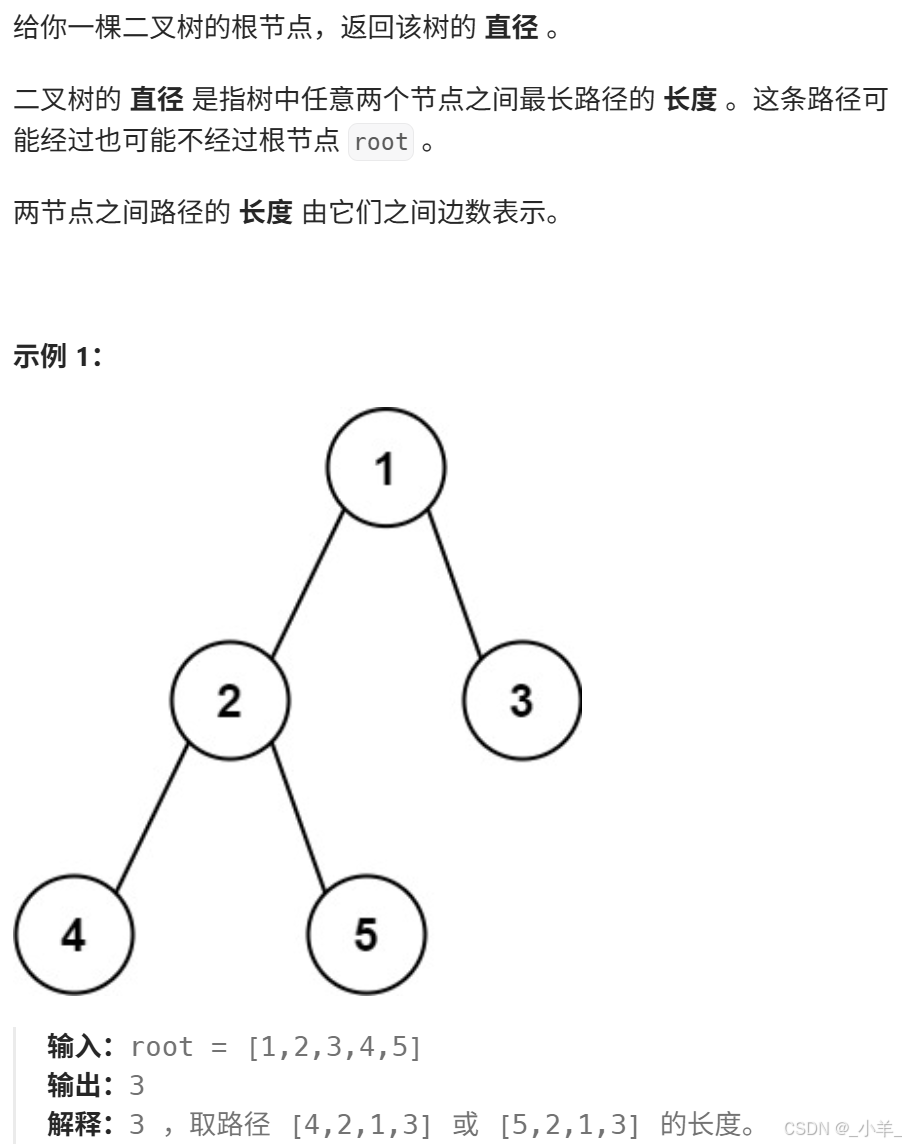
class Solution {int depth;
public:int diameterOfBinaryTree(TreeNode* root) {dfs(root);return depth - 1;}int dfs(TreeNode* root){if (root == nullptr) return 0;int left = dfs(root->left);int right = dfs(root->right);depth = max(depth, left + right + 1);return max(left, right) + 1;}
};
二叉树的层序遍历
- 二叉树的层序遍历
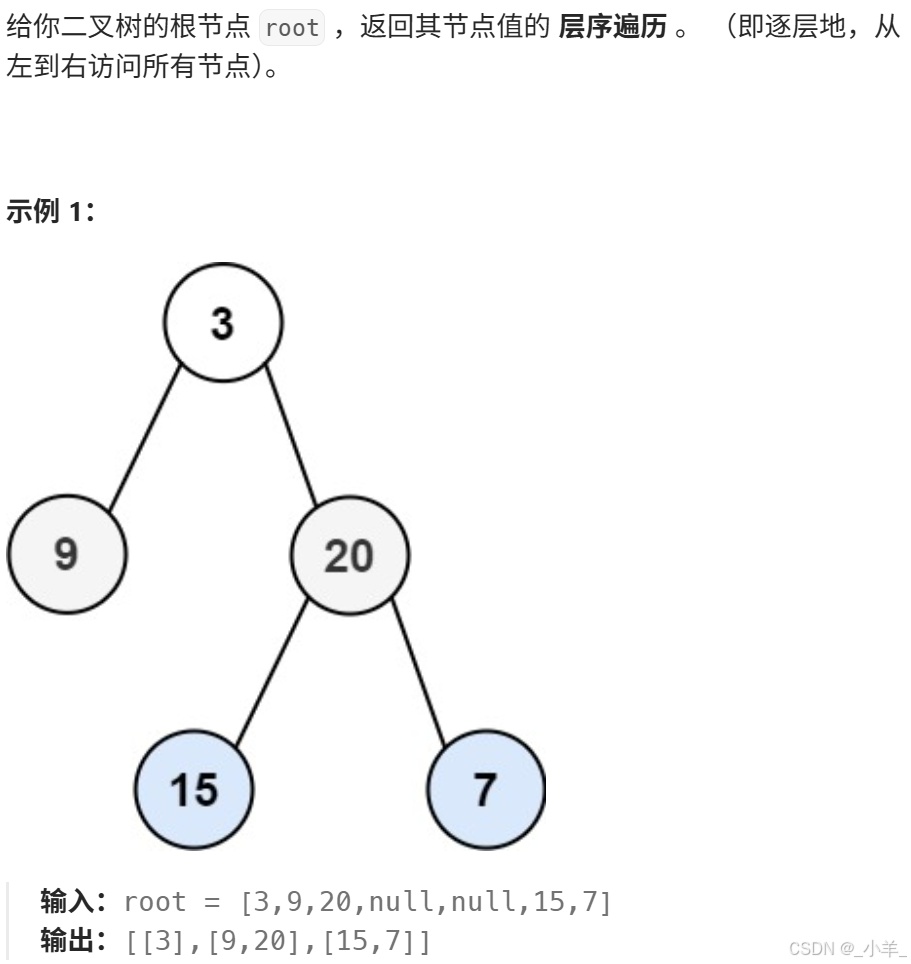
class Solution {
public:vector<vector<int>> levelOrder(TreeNode* root) {vector<vector<int>> res;queue<TreeNode*> q;if (root == nullptr) return res;q.push(root);while (q.size()){int sz = q.size();vector<int> tmp;while (sz--){TreeNode *node = q.front();tmp.push_back(node->val);q.pop();if (node->left) q.push(node->left);if (node->right) q.push(node->right);}res.push_back(tmp);}return res;}
};
将有序数组转换为二叉搜索树
- 将有序数组转换为二叉搜索树
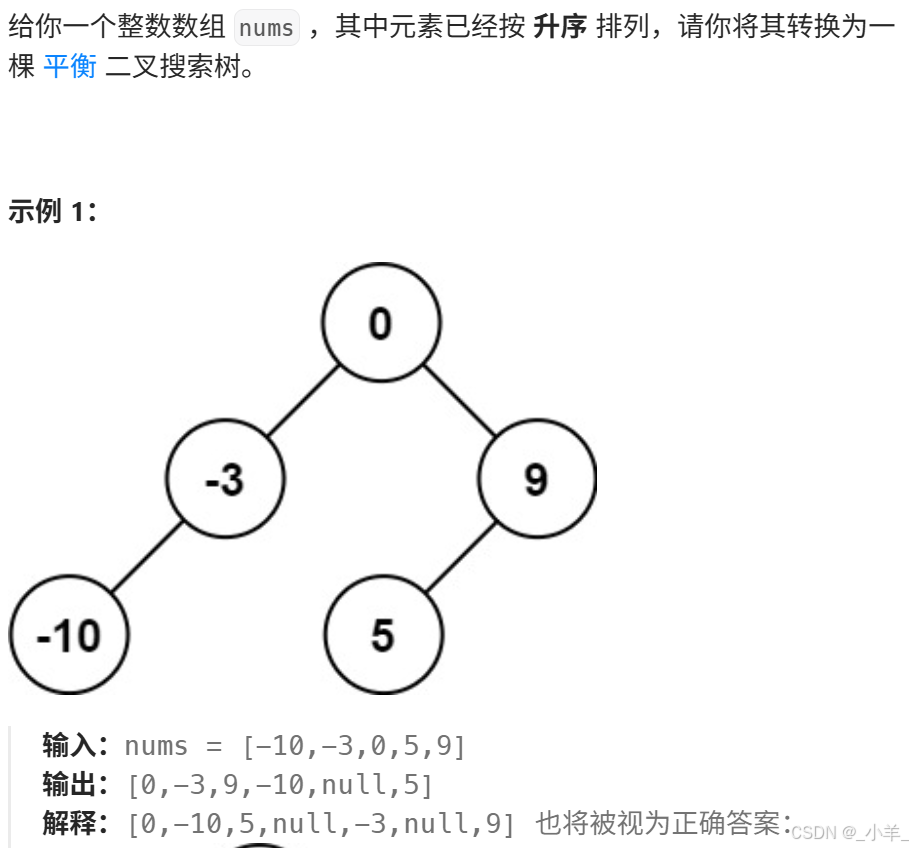
class Solution {
public:TreeNode* sortedArrayToBST(vector<int>& nums) {return dfs(nums, 0, nums.size() - 1);}TreeNode* dfs(vector<int>& nums, int l, int r){if (l > r) return nullptr;int mid = l + (r - l) / 2;TreeNode* node = new TreeNode(nums[mid]);node->left = dfs(nums, l, mid - 1);node->right = dfs(nums, mid + 1, r);return node;}
};
验证二叉搜索树
- 验证二叉搜索树
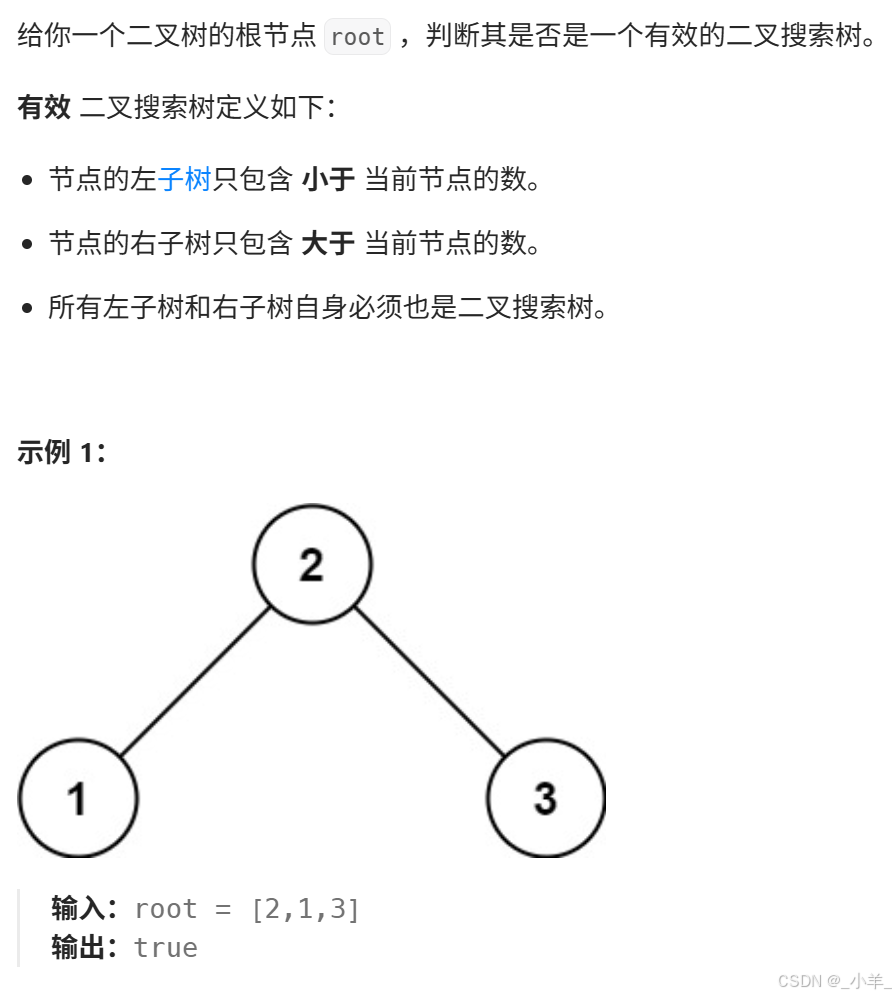
递归遍历。
class Solution {
public:bool isValidBST(TreeNode* root) {return dfs(root, LONG_MIN, LONG_MAX);}bool dfs(TreeNode* root, long min_val, long max_val){if (root == nullptr) return true;if (root->val <= min_val || root->val >= max_val) return false;return dfs(root->left, min_val, root->val) && dfs(root->right, root->val, max_val);}
};
前序遍历。
class Solution {long prev = LONG_MIN;
public:bool isValidBST(TreeNode* root) {if (root == nullptr) return true;if (isValidBST(root->left) == false) return false;if (root->val <= prev) return false;prev = root->val; return isValidBST(root->right);}
};
二叉搜索树中第 K 小的元素
- 二叉搜索树中第 K 小的元素
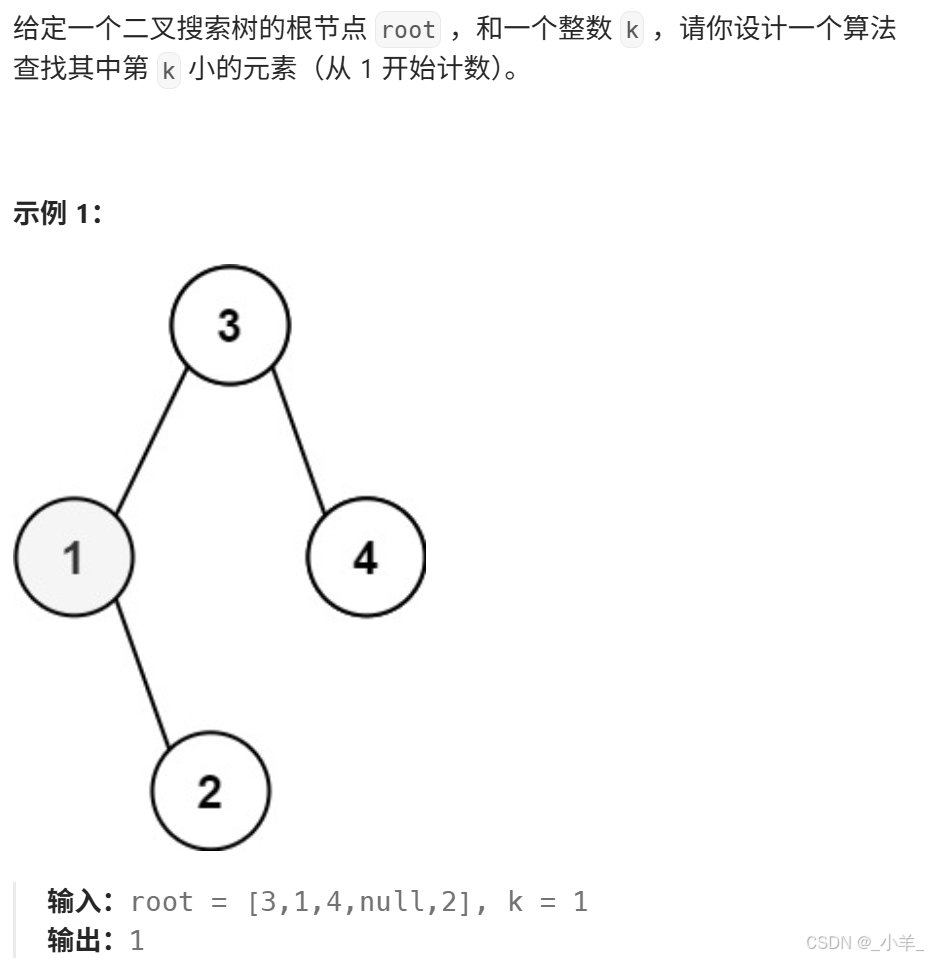
class Solution {int res, cnt;
public:int kthSmallest(TreeNode* root, int k) {cnt = k;dfs(root);return res;}void dfs(TreeNode* root){if (root == nullptr) return;dfs(root->left);if (--cnt == 0) {res = root->val;return;}dfs(root->right);}
};
二叉树的右视图
- 二叉树的右视图
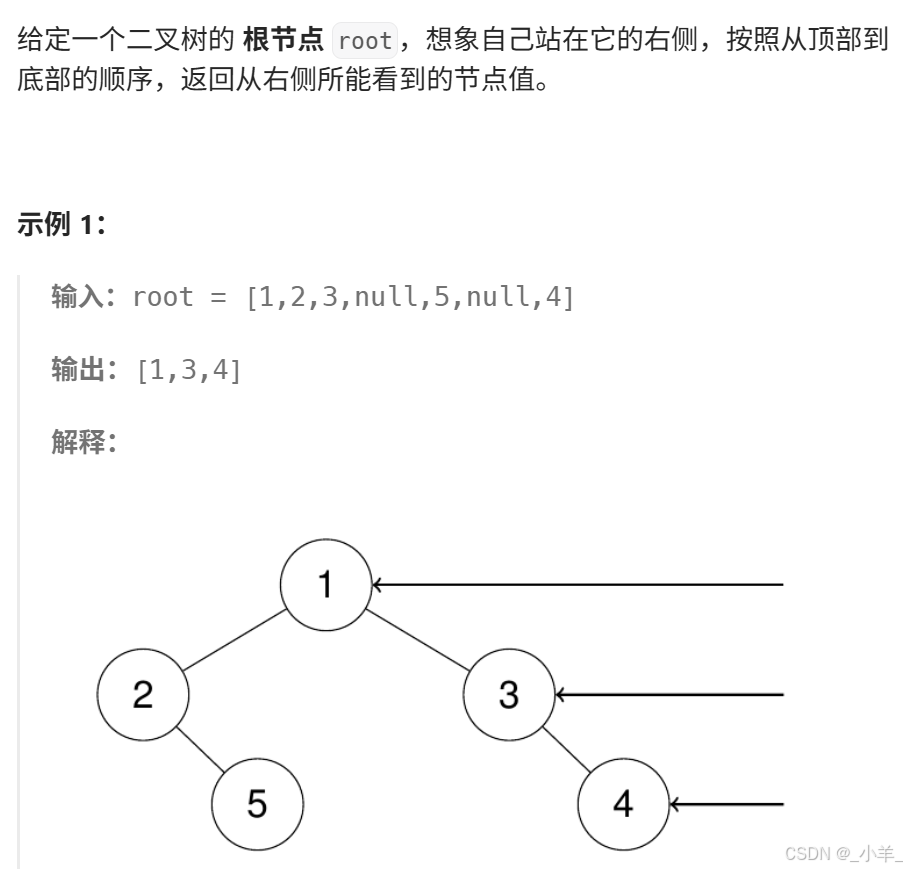
从右往左层序遍历,每次都只拿队头节点的值。
class Solution {
public:vector<int> rightSideView(TreeNode* root) {vector<int> res;if (root == nullptr) return res;queue<TreeNode*> q;q.push(root);while (q.size()){res.push_back(q.front()->val);int sz = q.size();while (sz--){TreeNode* node = q.front();q.pop();if (node->right) q.push(node->right);if (node->left) q.push(node->left);}}return res;}
};
二叉树展开为链表
- 二叉树展开为链表
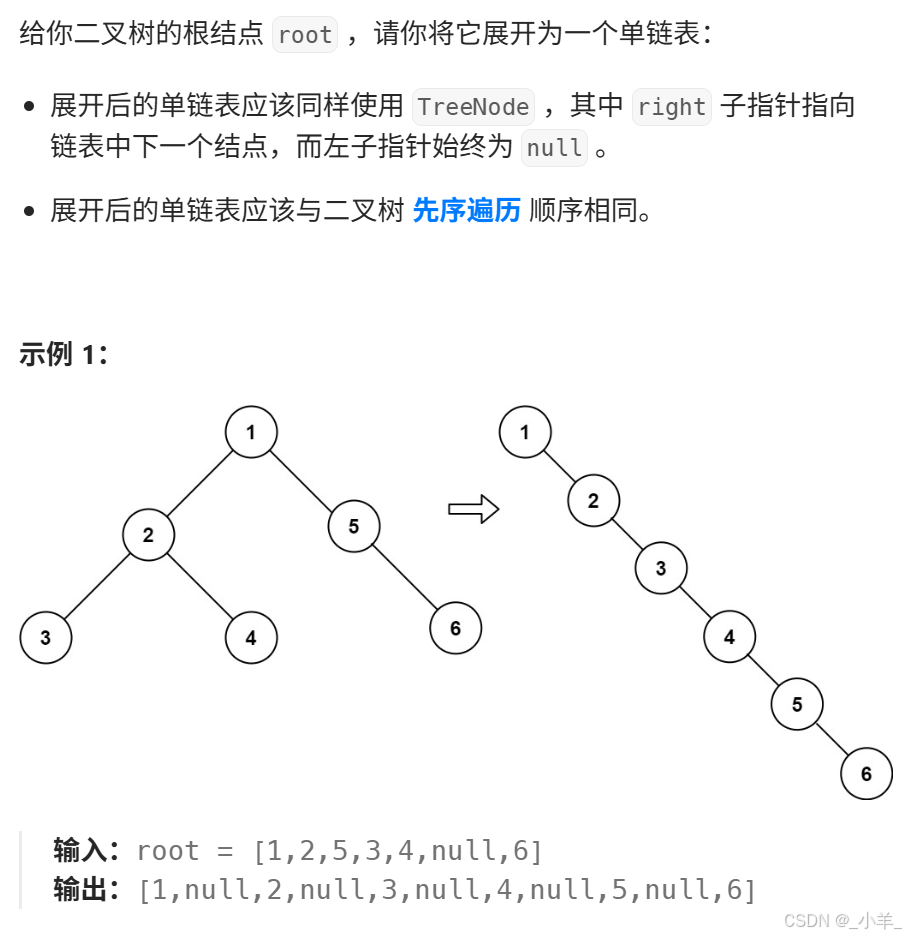
方法一:在前序遍历的过程中把节点存起来,结束后在处理每个节点的指向。
class Solution {
public:void flatten(TreeNode* root) {vector<TreeNode*> vt;dfs(root, vt);for (int i = 1; i < vt.size(); i++){TreeNode* prev = vt[i - 1], *cur = vt[i];prev->left = nullptr;prev->right = cur;}}void dfs(TreeNode* root, vector<TreeNode*>& vt){if (root){vt.push_back(root);dfs(root->left, vt);dfs(root->right, vt);}}
};
方法二:寻找前驱结点法。
在前序遍历的过程中,如果当前节点的左子树不为空,则遍历到当前节点的右子树的前一个节点为:当前节点左子树中最右的那个节点。我们在遍历的过程中找到这个前驱结点,将当前节点的右子树拼接到这个前驱节点的右节点上,然后将当前节点的左子树拼接到当前节点的右子树上,最后当前节点左子树置空。
class Solution {
public:void flatten(TreeNode* root) {TreeNode* cur = root;while (cur){if (cur->left){auto prev = cur->left;while (prev->right) prev = prev->right;prev->right = cur->right;cur->right = cur->left;cur->left = nullptr;}cur = cur->right;}}
};
从前序与中序遍历序列构造二叉树
- 从前序与中序遍历序列构造二叉树

根据前序遍历依次找到根节点,通过找到的根节点找到中序遍历中根节点的位置,从而划分出 [左子树] [根节点] [右子树]。
用哈希表存储中序遍历的值和下标映射关系,以至于能在找到根节点后直接拿到根节点在中序遍历中的位置,然后根据中序遍历的位置递归构建左子树和右子树。
class Solution {unordered_map<int, int> index;
public:TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {for (int i = 0; i < inorder.size(); i++){index[inorder[i]] = i;}return dfs(preorder, 0, 0, inorder.size() - 1);}TreeNode* dfs(vector<int>& preorder, int root, int l, int r){if (l > r) return nullptr;TreeNode* node = new TreeNode(preorder[root]);int id = index[preorder[root]];node->left = dfs(preorder, root + 1, l, id - 1);node->right = dfs(preorder, root + id - l + 1, id + 1, r);return node;}
};
路径总和
- 路径总和
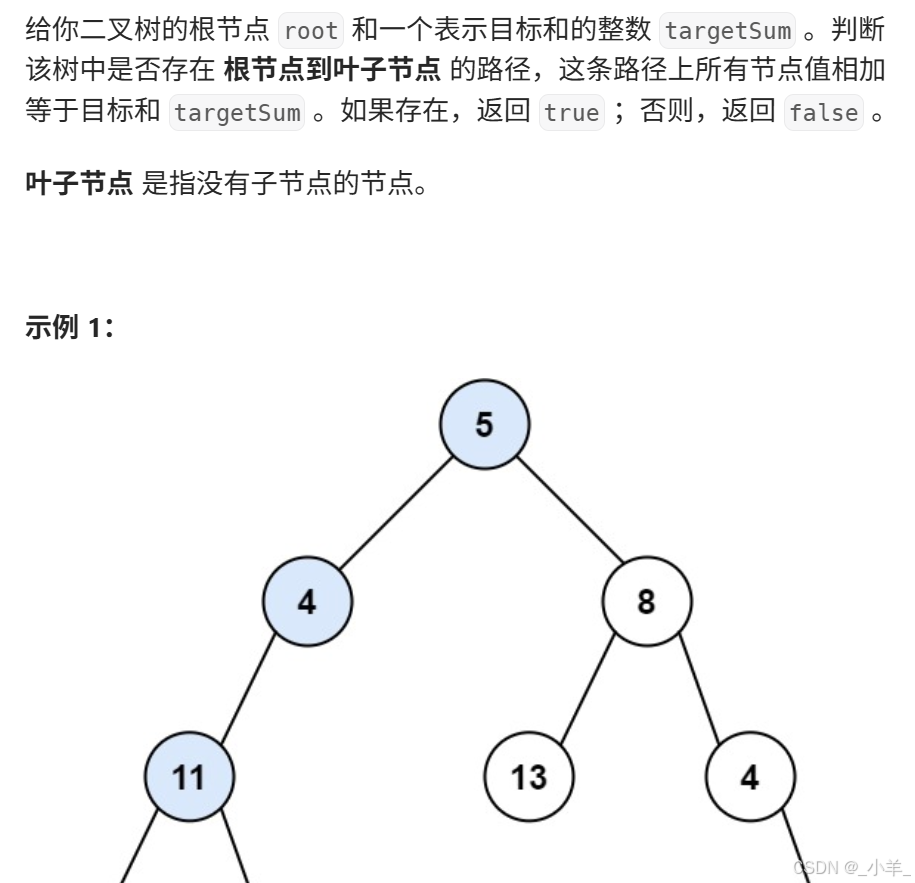
class Solution {
public:bool hasPathSum(TreeNode* root, int targetSum) {if (root == nullptr) return false;if (root->left == nullptr && root->right == nullptr) {return targetSum == root->val;}return hasPathSum(root->left, targetSum - root->val) || hasPathSum(root->right, targetSum - root->val);}
};
路径总和 II
- 路径总和 II
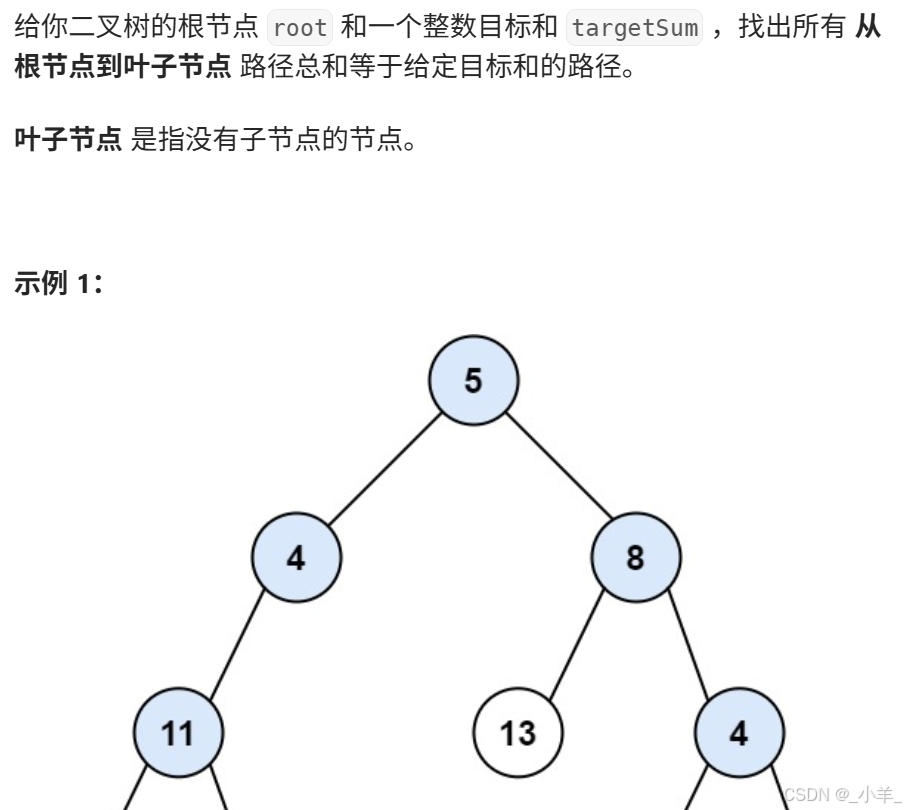
class Solution {vector<vector<int>> res;vector<int> path;
public:vector<vector<int>> pathSum(TreeNode* root, int targetSum) {dfs(root, targetSum);return res;}void dfs(TreeNode* root, int t){if (root == nullptr) return;path.push_back(root->val);if (root->left == nullptr && root->right == nullptr && t == root->val){res.push_back(path);}dfs(root->left, t - root->val);dfs(root->right, t - root->val);path.pop_back(); // 回溯}
};
路径总和 III
- 路径总和 III
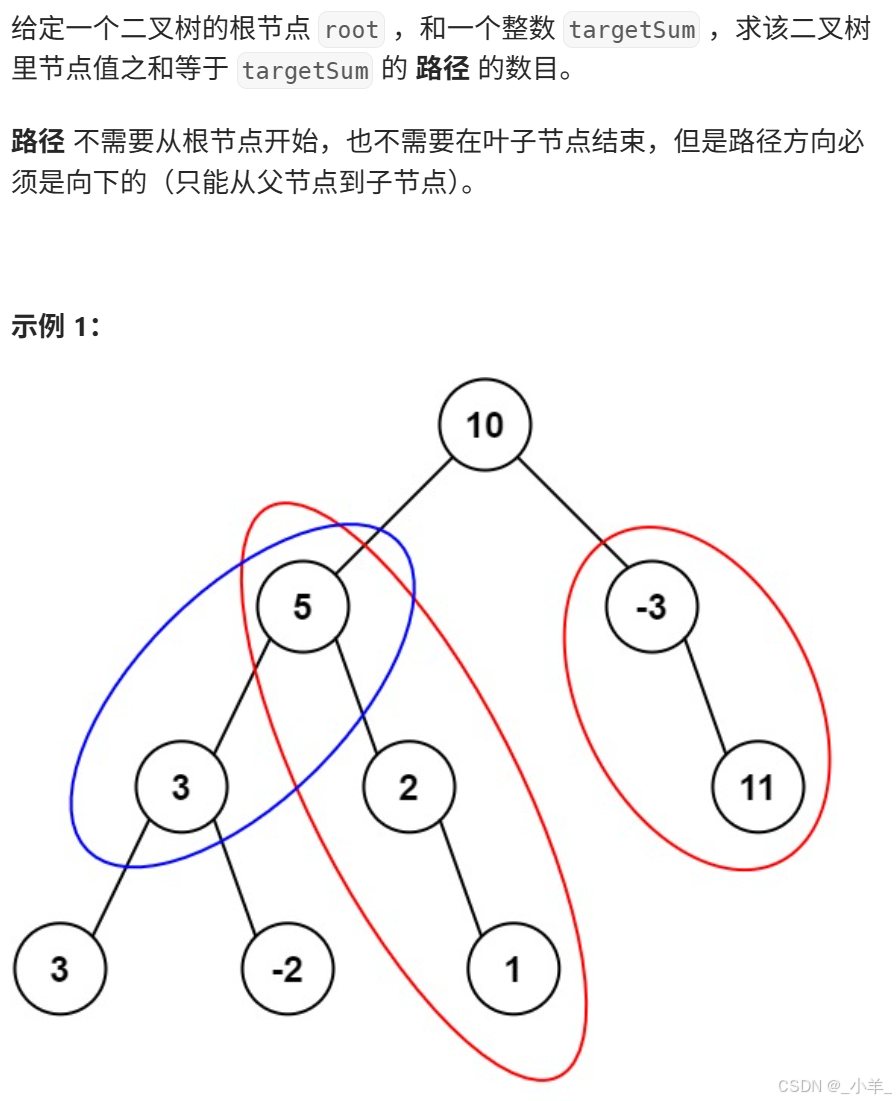
class Solution {using ll = long long;unordered_map<ll, int> pre_cnt; // 记录前缀和及其出现次数int t;
public:int pathSum(TreeNode* root, int targetSum) {t = targetSum;pre_cnt[0] = 1; // 前缀和恰好等于目标值return dfs(root, 0);}int dfs(TreeNode* root, ll sum) {if (root == nullptr) return 0;sum += root->val;int count = pre_cnt[sum - t];pre_cnt[sum]++;count += dfs(root->left, sum);count += dfs(root->right, sum);pre_cnt[sum]--;return count;}
};
本篇文章的分享就到这里了,如果您觉得在本文有所收获,还请留下您的三连支持哦~


LLM数据基础-分词算法(2))


Python爬虫高阶:动态页面处理与云原生部署全链路实践(Selenium、Scrapy、K8s))
——排序)




View相关知识)



发布)




