---

前言

最短路Dijkstra，spfa，图论二分图算法AYIT—ACM训练(模板版)
A — Dijkstra
B — spfa/Dijkstra
C — 二分图
D — 二分图
E — 二分图
F — 二分图
G — Dijkstra
H — Topsort

---

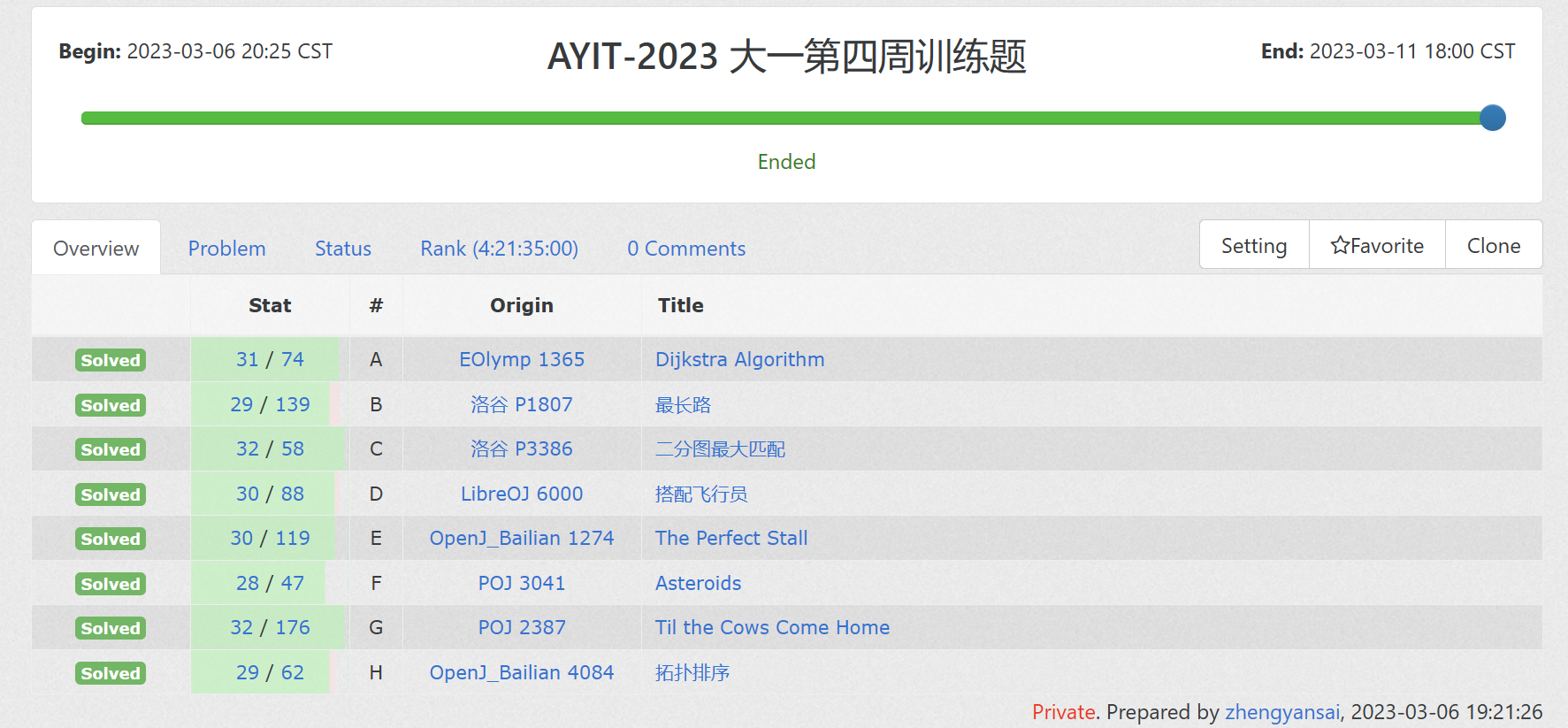
A - Dijkstra Algorithm

0x00 算法题目

0x01 算法思路

Dijkstra算法基础模板题

💬 模板演示：

int dijkstra()
{memset(dist,0x3f,sizeof dist);dist[1]=0;for(int i=0;i<n;i++){int t=-1;for(int j=1;j<=n;j++){if(!st[j] && (t==-1 || dist[t] > dist[j]))t=j;}st[t]=true;for(int j=1;j<=n;j++)dist[j]=min(dist[j],dist[t]+g[t][j]);}if(dist[n]==0x3f3f3f3f) return -1;return dist[n];}

0x02 代码实现

朴素版本Dijkstra：

💬 代码演示：

#include<iostream>
#include<cstring>
#include<algorithm>using namespace std;
const int N = 510;
int g[N][N];
bool st[N];
int dist[N];
int n,s,f;int dijkstra()
{memset(dist,0x3f,sizeof dist);dist[s]=0;for(int i=0;i<n;i++){int t=-1;for(int j=1;j<=n;j++)if(!st[j] && (t==-1 || dist[t] > dist[j]))t=j;st[t]=true;for(int j=1;j<=n;j++)dist[j]=min(dist[j],dist[t]+g[t][j]);}if(dist[f]==0x3f3f3f3f) return -1;return dist[f];
}int main()
{cin>>n>>s>>f;for(int i=1;i<=n;i++){for(int j=1;j<=n;j++){int x;cin>>x;if(x==-1) g[i][j]=0x3f3f3f3f;else g[i][j]=x;}}int t =dijkstra();cout<<t<<endl;return 0;
}

🚩 运行结果：

spfa算法：
💬 代码演示：

#include<iostream>
#include<cstring>
#include<algorithm>
#include<queue>using namespace std;
const int N=110,M=110*110;
int n,s,f;
bool st[N];
int h[N],w[M],ne[M],e[M],idx;
int dist[N];void add(int a,int b,int c)
{e[idx]=b,w[idx]=c,ne[idx]=h[a],h[a]=idx++;
}int spfa()
{memset(dist,0x3f,sizeof dist);dist[s]=0;queue<int> q;q.push(s);while(q.size()){int t = q.front();q.pop();st[t]=false;for(int i=h[t];i!=-1;i=ne[i]){int j=e[i];if(dist[j] > dist[t] + w[i]){dist[j]=dist[t]+w[i];if(!st[j]){q.push(j);st[j]=true;}}}}if(dist[f]==0x3f3f3f3f) return -1;else return dist[f];
}int main()
{cin>>n>>s>>f;memset(h,-1,sizeof h);for(int i=1;i<=n;i++){for(int j=1;j<=n;j++){int x;cin>>x;//if(x==-1) continue;if(x>0) add(i,j,x);}}cout<<spfa()<<endl;return 0;
}

🚩 运行结果：

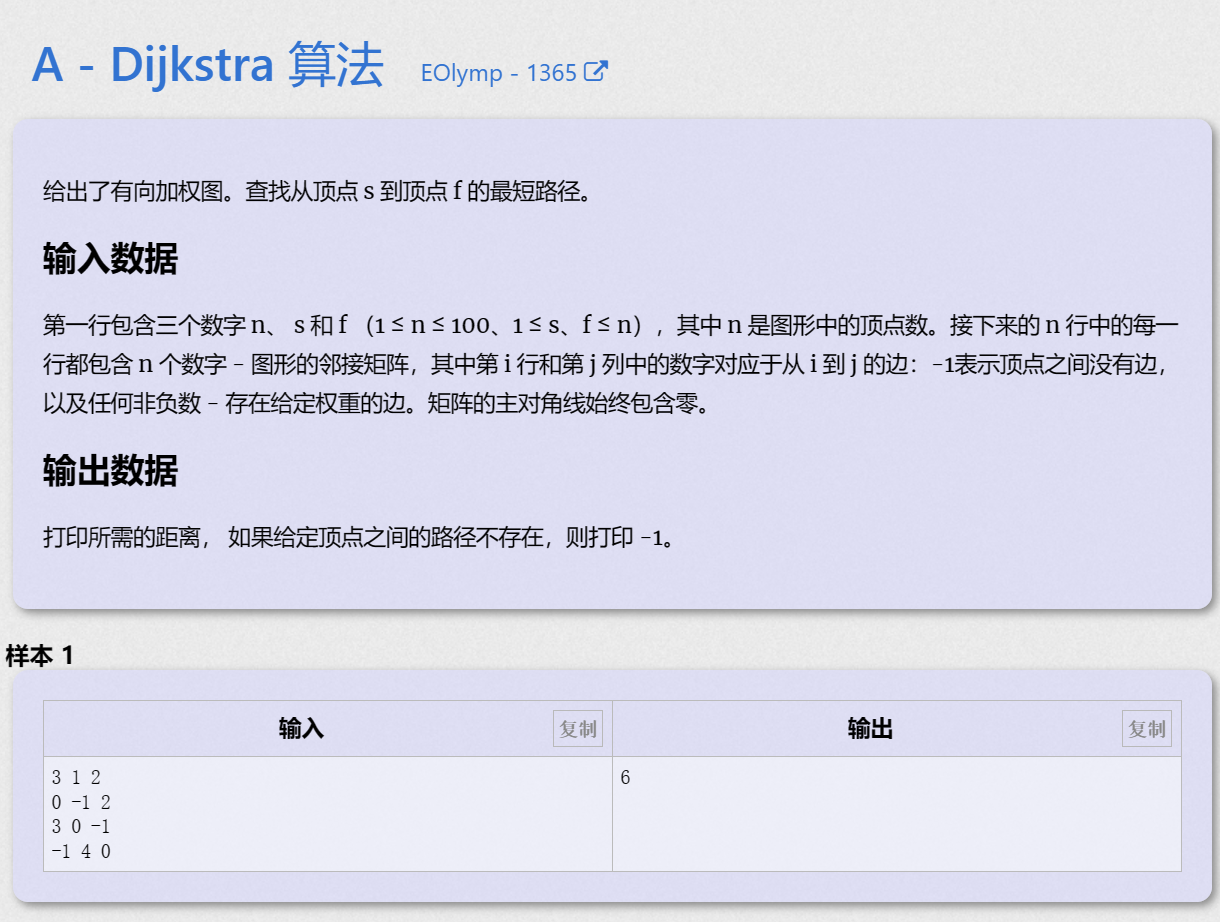
B - 最长路

0x00 算法题目

0x01 算法思路

spfa算法基础模板题

💬 模板演示：

int spfa()
{memset(dist, 0x3f, sizeof dist);dist[1] = 0;queue<int> q;q.push(1);while(q.size()){auto t = q.front();q.pop();st[t]=false;for(int i=h[t];i!=-1;i=ne[i]){int j = e[i];if(dist[j] > dist[t]+w[i]){dist[j]=dist[t]+w[i];if(!st[j]){q.push(j);st[j]=true;}}}}return dist[n];
}

0x02 代码实现

spfa算法：
💬 代码演示：

#include<bits/stdc++.h>
#define endl '\n'using namespace std;
const int N = 1510,INF = 0x3f3f3f3f;
int n,m;
int dist[N];
int g[N][N];
queue<int> q;void spfa()
{memset(dist,-1,sizeof dist);dist[1]=0;q.push(1);while(!q.empty()){int t = q.front();q.pop();for(int j=1;j<=n;j++){if(g[t][j] && dist[j] < dist[t] + g[t][j]){dist[j] = dist[t] + g[t][j];q.push(j);}}}}int main()
{cin>>n>>m;for(int i=1;i<=m;i++){int a,b,c;cin>>a>>b>>c;g[a][b]=max(g[a][b],c);}spfa();cout<<dist[n]<<endl;return 0;
}

🚩 运行结果：

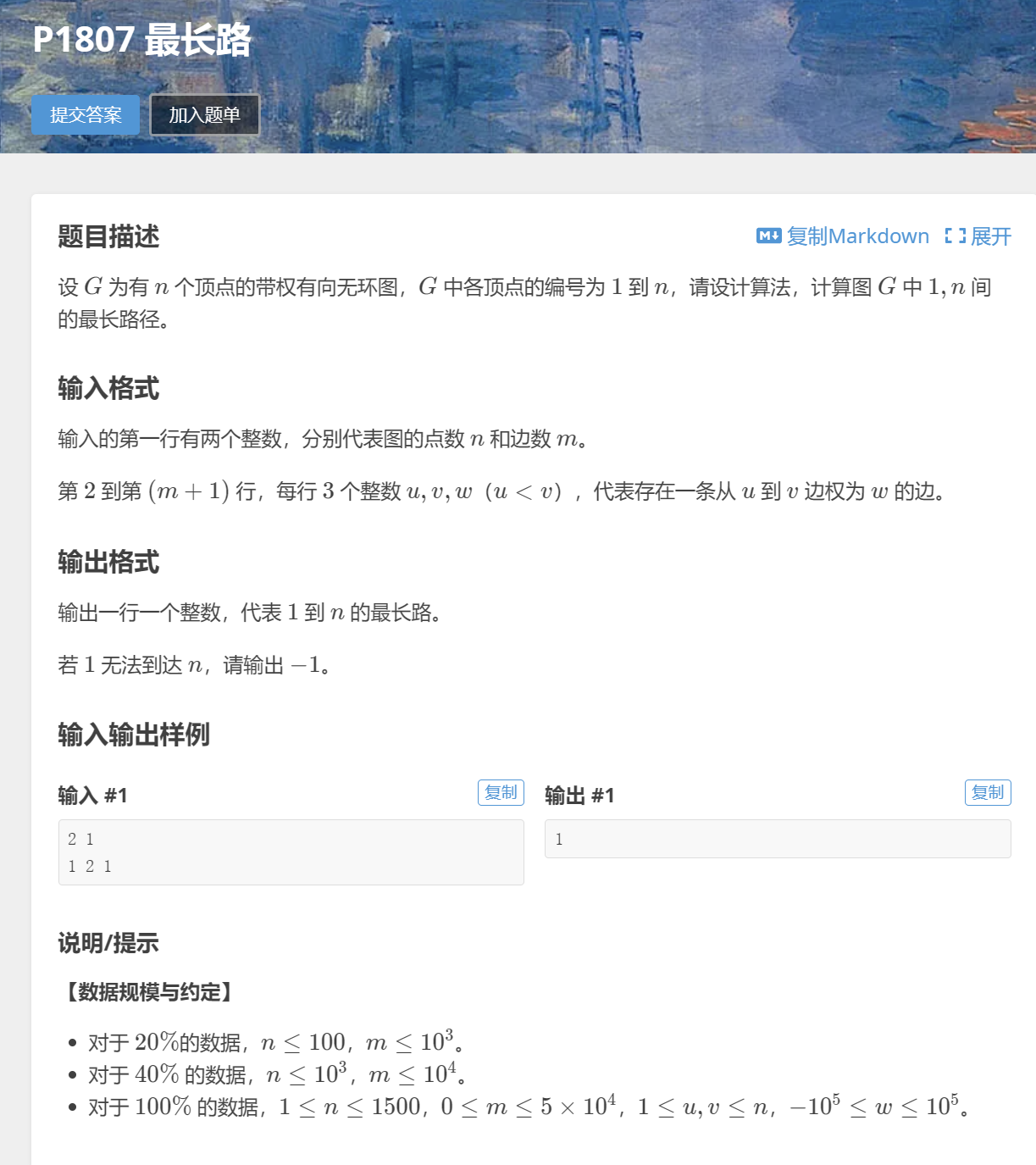
C - 二分图最大匹配

0x00 算法题目

0x01 算法思路

二分图模板题

💬 模板演示：

//邻接表
bool find(int x)
{for (int i = h[x]; i != -1; i = ne[i]){int j = e[i];if (!st[j]){st[j] = true;if (match[j] == 0 || find(match[j])){match[j] = x;return true;}}}return false;
}
//邻接矩阵
bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}

0x02 代码实现

💬 代码演示：

#include<iostream>
#include<cstring>using namespace std;const int N = 510,M=5e4+10;
int n,m,q;
int h[N],e[M],ne[M],idx;
int match[N];
bool st[N];void add(int a,int b)
{e[idx]=b,ne[idx]=h[a],h[a]=idx++;
}bool find(int x)
{for(int i=h[x];i!=-1;i=ne[i]){int j = e[i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}int main()
{cin>>n>>m>>q;memset(h,-1,sizeof h);while(q--){int u,v;cin>>u>>v;add(u,v);}int res=0;for(int i=1;i<=n;i++){memset(st,false,sizeof st);if(find(i)) res++;}cout<<res<<endl;return 0;
}

🚩 运行结果：

D - 搭配飞行员

0x00 算法题目

0x01 算法思路

二分图模板题

💬 模板演示：

//邻接表
bool find(int x)
{for (int i = h[x]; i != -1; i = ne[i]){int j = e[i];if (!st[j]){st[j] = true;if (match[j] == 0 || find(match[j])){match[j] = x;return true;}}}return false;
}
//邻接矩阵
bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}

0x02 代码实现

💬 代码演示：

#include<iostream>
#include<cstring>
#include<algorithm>
#include<bits/stdc++.h>using namespace std;
const int N = 110;int n,m;
int map[N][N];
int match[N];
bool st[N];
vector<int> g[N];
bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}int main()
{scanf("%d %d",&n,&m);int a,b;while(cin>>a>>b){g[a].push_back(b);}int res = 0;for(int i=1;i<=m;i++){memset(st,false,sizeof st);if(find(i)) {res++;}}cout<<res;return 0;
}

🚩 运行结果：

E - The Perfect Stall

0x00 算法题目

0x01 算法思路

二分图模板题

💬 模板演示：

//邻接表
bool find(int x)
{for (int i = h[x]; i != -1; i = ne[i]){int j = e[i];if (!st[j]){st[j] = true;if (match[j] == 0 || find(match[j])){match[j] = x;return true;}}}return false;
}
//邻接矩阵
bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}

0x02 代码实现

💬 代码演示：

#include<algorithm>
#include<bits/stdc++.h>
using namespace std;
const int N = 510,M=5e4+10;
int n,m;
int match[N];
bool st[N];
vector<int> g[N];bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}int main()
{while(~scanf("%d%d",&n,&m)){memset(st,false,sizeof st);memset(match,0,sizeof match);for(int i=1;i<=n;i++){g[i].clear();int s;cin>>s;while(s--){int q;cin>>q;g[i].push_back(q);}}int res=0;for(int i=1;i<=n;i++){memset(st,false,sizeof st);if(find(i)) res++;}cout<<res<<endl;}return 0;
}

🚩 运行结果：

F - Asteroids

0x00 算法题目

0x01 算法思路

二分图模板题

💬 模板演示：

//邻接表
bool find(int x)
{for (int i = h[x]; i != -1; i = ne[i]){int j = e[i];if (!st[j]){st[j] = true;if (match[j] == 0 || find(match[j])){match[j] = x;return true;}}}return false;
}
//邻接矩阵
bool find(int x)
{for(int i=0;i<g[x].size();++i){int j = g[x][i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}

0x02 代码实现

💬 代码演示：

#include<iostream>
#include<cstring>
using namespace std;
const int N = 510,M=5e4+10;
int n,m,q;
int h[N],e[M],ne[M],idx;
int match[N];
bool st[N];void add(int a,int b)
{e[idx]=b,ne[idx]=h[a],h[a]=idx++;
}bool find(int x)
{for(int i=h[x];i!=-1;i=ne[i]){int j = e[i];if(!st[j]){st[j]=true;if(match[j]==0 || find(match[j])){match[j]=x;return true;}}}return false;
}int main()
{cin>>n>>m;memset(h,-1,sizeof h);while(m--){int u,v;cin>>u>>v;add(u,v);}int res=0;for(int i=1;i<=n;i++){memset(st,false,sizeof st);if(find(i)) res++;}cout<<res<<endl;return 0;
}

🚩 运行结果：

G - Til the Cows Come Home

0x00 算法题目

0x01 算法思路

Dijkstra算法基础模板题

💬 模板演示：

int dijkstra()
{memset(dist,0x3f,sizeof dist);dist[1]=0;for(int i=0;i<n;i++){int t=-1;for(int j=1;j<=n;j++){if(!st[j] && (t==-1 || dist[t] > dist[j]))t=j;}st[t]=true;for(int j=1;j<=n;j++)dist[j]=min(dist[j],dist[t]+g[t][j]);}if(dist[n]==0x3f3f3f3f) return -1;return dist[n];}

0x02 代码实现

💬 代码演示：

#include<iostream>
#include<cstring>
#include<algorithm>
#include<stdbool.h>using namespace std;const int N=1010,inf = 0x3f3f3f3f;int n,m;
bool st[N];
int dist[N];
int g[N][N];int dijkstra()
{memset(dist,inf,sizeof(dist));dist[1]= 0;for(int i=1;i <= n;i++){int t=-1;for(int j=1;j<=n;j++)if(!st[j] && (t==-1 || dist[t] > dist[j]))t=j;st[t]=true;for(int j=1;j<=n;j++)dist[j]=min(dist[j],dist[t]+g[t][j]);}return dist[n];
}int main()
{cin>>m>>n;memset(g,inf,sizeof g);for(int i=0;i<m;++i){int a,b,c;cin>>a>>b>>c; g[a][b]=g[b][a]=min(g[a][b],c);}cout<< dijkstra() <<endl;return 0;
}

🚩 运行结果：

H - 拓扑排序

0x00 算法题目

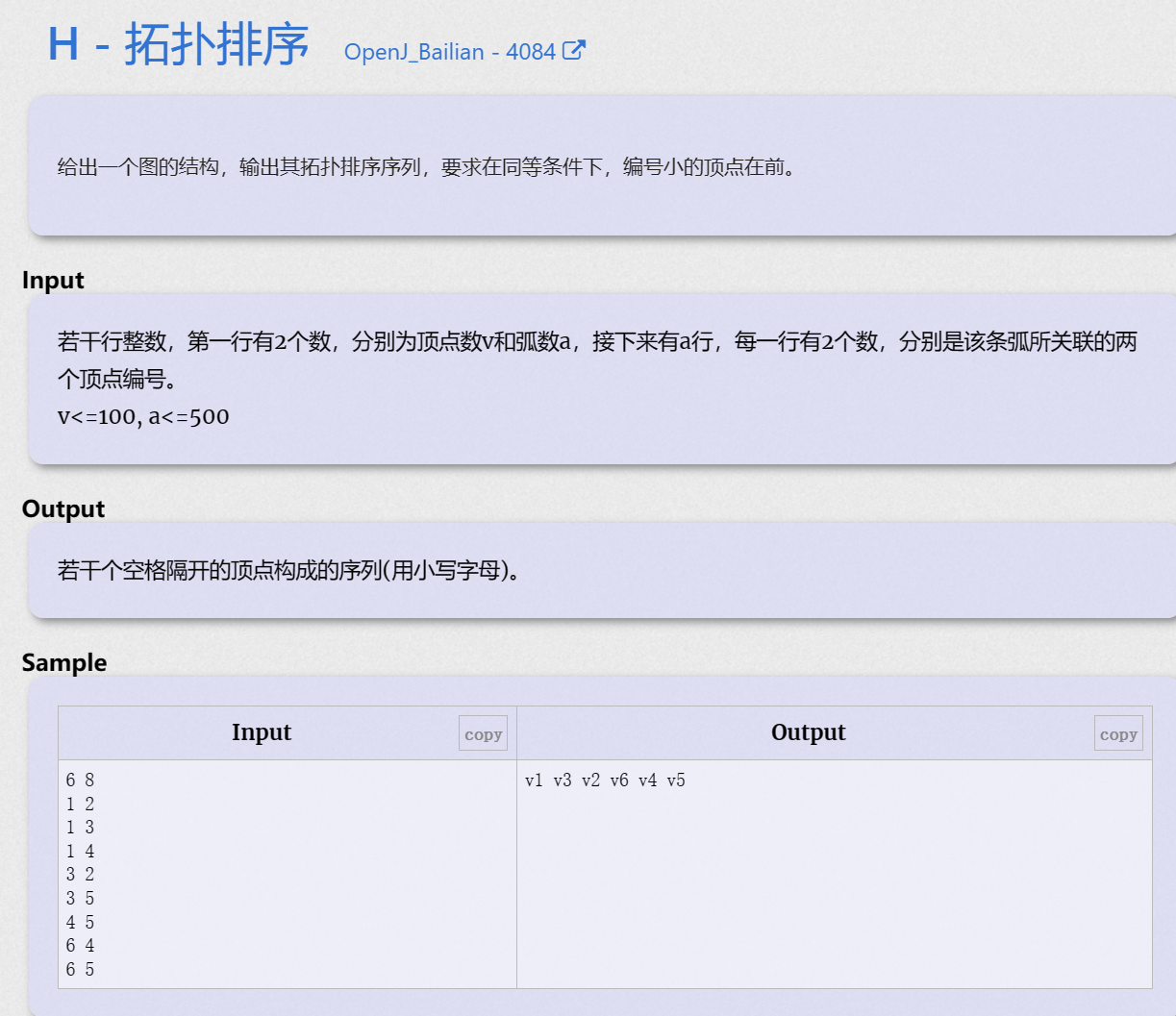
0x01 算法思路

拓扑排序算法基础模板题

💬 模板演示：

bool topsort()
{int hh=0,tt=-1;for (int i = 1; i <= n; i ++ )if (!d[i])q[ ++ tt] = i;while(hh<=tt){int t = q[hh ++ ];for (int i = h[t]; i != -1; i = ne[i]){int j = e[i];if (-- d[j] == 0)q[ ++ tt] = j;}}return tt==n-1;
}

0x02 代码实现

💬 代码演示：

#include<iostream>
#include<cstring>
#include<algorithm>
#include<queue>
#include<vector>using namespace std;const int N=100010;int n,m;
vector<int> v[N];
int size,d[N];
int ans[N];void topsort()
{priority_queue<int,vector<int>,greater<int> > q;for(int i=1;i<=n;i++)if(!d[i]) q.push(i);while(!q.empty()){int t = q.top();q.pop();ans[size++] = t;for(auto it : v[t]){d[it]--;if(!d[it]) q.push(it);}}
}int main()
{cin>>n>>m;for(int i=0;i<m;i++){int a,b;cin>>a>>b;v[a].push_back(b);d[b]++;}topsort();for(int i=0 ; i<size ;i++)cout<< 'v' << ans[i] <<' ';return 0;
}

🚩 运行结果：

---

总结

这次训练很明显涉及到了最短路Dijkstra，spfa，图论二分图算法，以及topsort算法，这次考的比较基础，但是让我意识到了，算法必须熟练记忆模板是很重要的。